S. Remmler, S. Hickel, M.D. Fruman, U. Achatz (2015)
Journal of the Atmospheric Sciences 72: 3537-3562. doi: 10.1175/JAS-D-14-0321.1
To reduce the computational costs of numerical studies of gravity wave breaking in the atmosphere, the grid resolution has to be reduced as much as possible. Insufficient resolution of small-scale turbulence demands a proper turbulence parameterization in the framework of a large-eddy simulation (LES). We consider three different LES methods—the adaptive local deconvolution method (ALDM), the dynamic Smagorinsky method (DSM), and a naïve central discretization without turbulence parameterization (CDS4)—for three different cases of the breaking of well-defined monochromatic gravity waves.
For ALDM, a modification of the numerical flux functions is developed that significantly improves the simulation results in the case of a temporarily very smooth velocity field. The test cases include an unstable and a stable inertia–gravity wave as well as an unstable high-frequency gravity wave. All simulations are carried out both in three-dimensional domains and in two-dimensional domains in which the velocity and vorticity fields are three-dimensional (so-called 2.5D simulations). The results obtained with ALDM and DSM are generally in good agreement with the reference direct numerical simulations as long as the resolution in the direction of the wave vector is sufficiently high. The resolution in the other directions has a weaker influence on the results. The simulations without turbulence parameterization are only successful if the resolution is high and the level of turbulence is comparatively low.
–
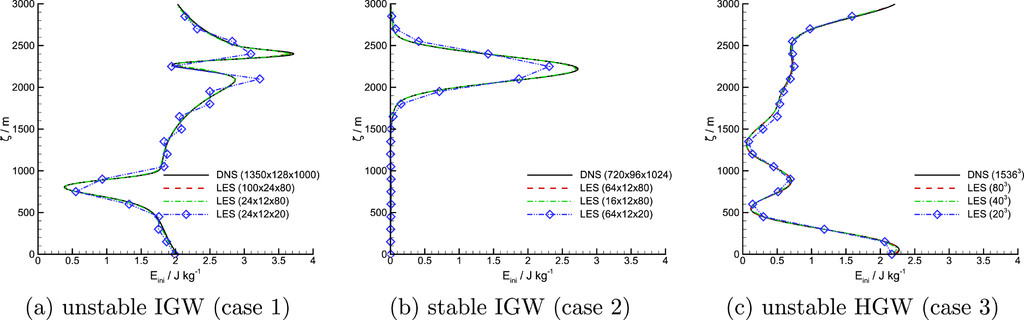
) averaged perturbation energy at different 3D grid resolutions.